Big Idea 2 Quiz
Below are the questions I had trouble with:
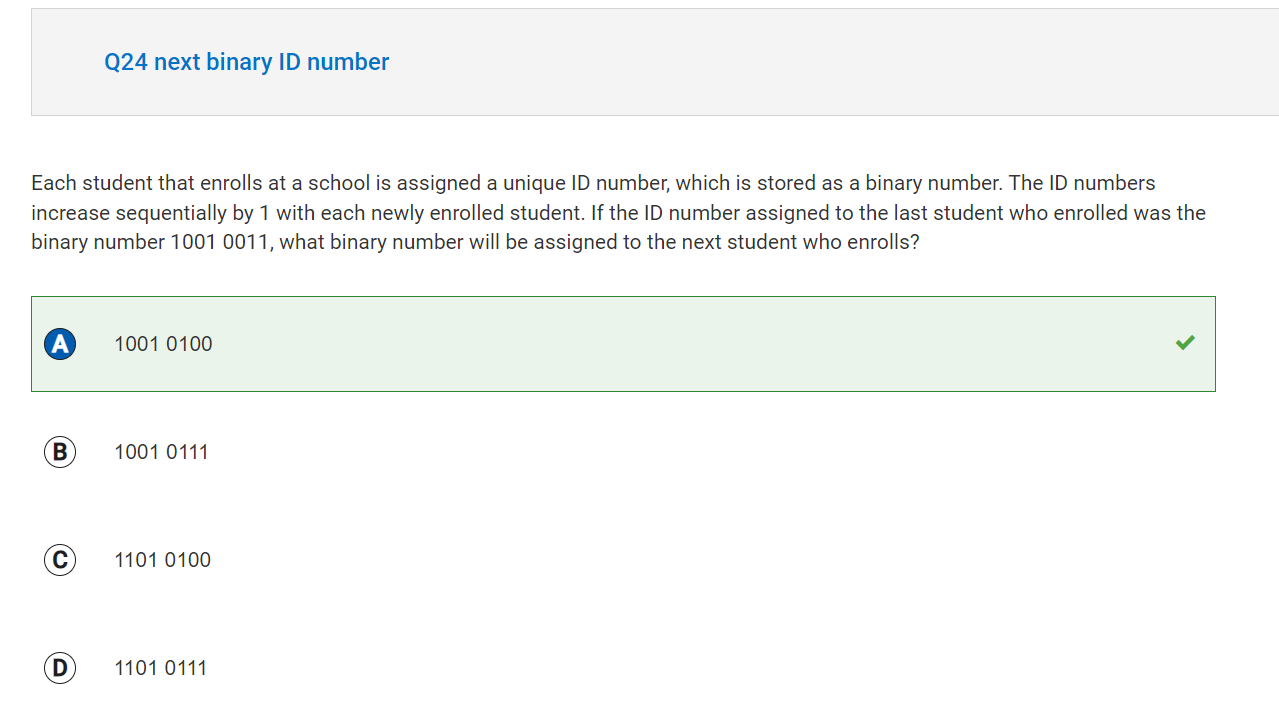
Question 1: At first, I was confused and didn’t know how to start the question. However, I remembered that the number of differet binary numbers is 2 to the power of bits. Since 75-100 items were needed, 2^7 = 7 bits = 128 numbers satisfies the requirement.
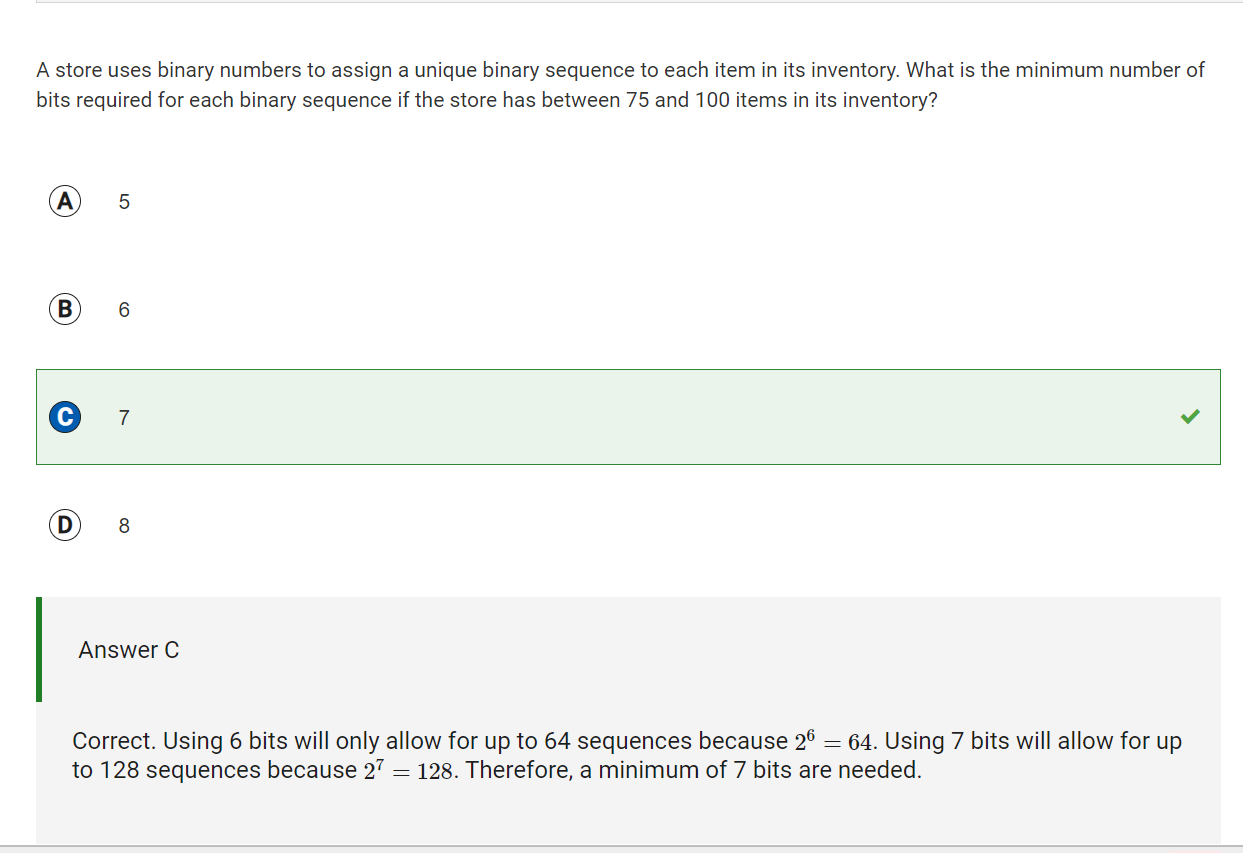
Question 7: At first, I thought that any of the answers could be correct because it is possible for two students to have the same name, gpa, grade level, or number of absences. However, since students are identified by name, having ids would solve the problem of students with the same name getting confused with each other.
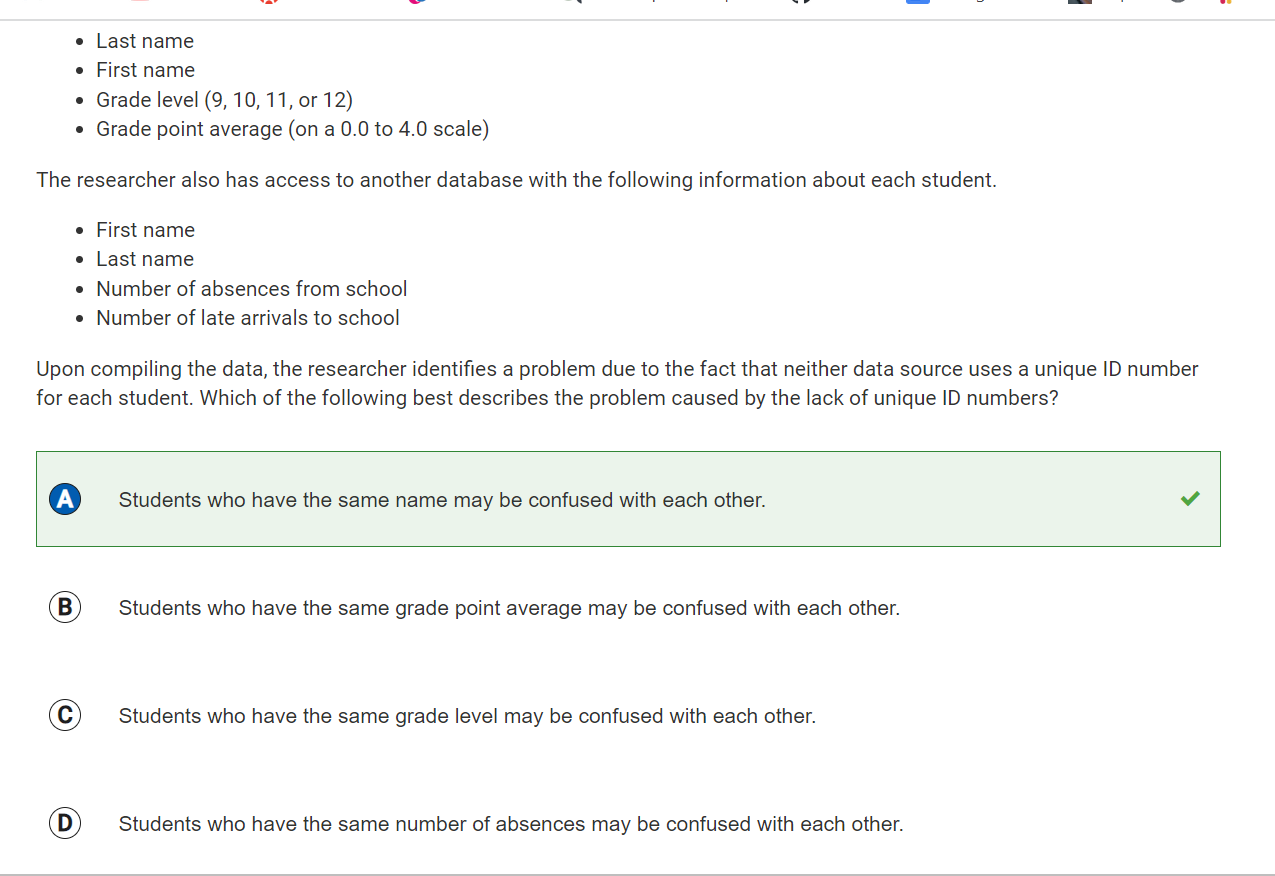
Question 24: At first, I only knew how to do this question by converting to decimal, adding 1, and converting back to binary. However, I figured out that adding 1 to each bit, unless that digit is a one, turn it into a 0 then add 1 to the next bit, and so on.